Vertical speeds on non-standard gradients
Many of us are familiar with the rule that your vertical speed on a 3-degree glideslope should be GS*5, e.g. at 100 knots you need 500 FPM. Captain Eddie has a good explanation of why it works. The only problem is that this doesn't work for other glide path angles that we increasingly see on some of the newer RNAV approaches, so we need a more flexible rule. As with the turn-in rule, we can lean on the fact that at small angles, the change in angle and the change of the tangent of that angle are fairly proportional (any engineers please take a deep breath), at least to the precision required to fly well. Let's take an example and use that as a basis.
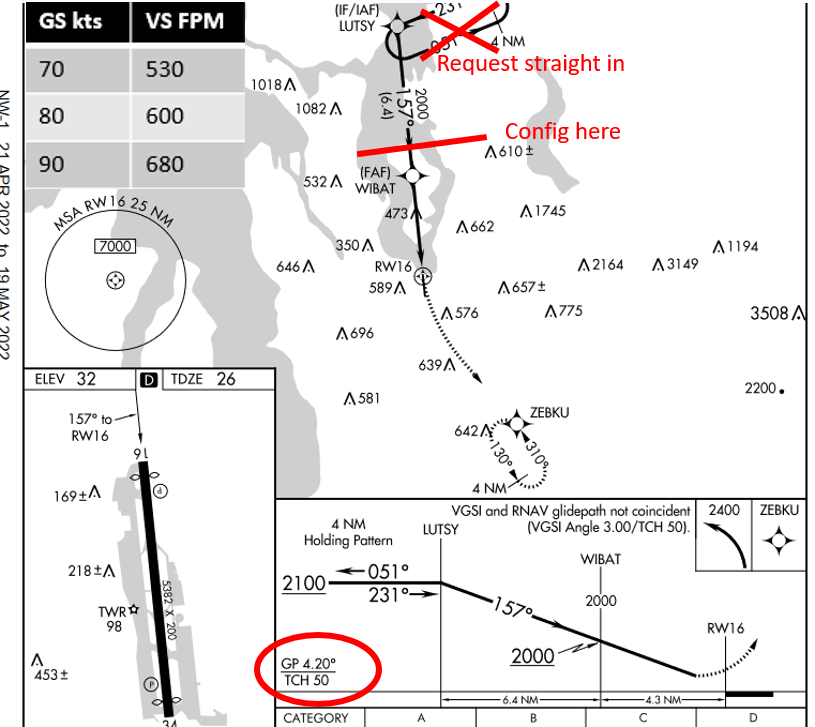
One approach that catches some people off guard on the descent is the RNAV 16Z into Renton. It's got a 4.2 degree glide path, so any time someone's coming in and pulls power to where they'd want it for a 3-degree path, they're going to end up high, and then sometimes chase the diamond to get back on glide. With that steep approach, that can mean exceeding 1000 FPM in the descent. If we use the equation VS=tan(GPA)*GS, we can figure out what our VS should be, and then make a rule. We need to convert from knots to FPM, which means multiplying by 6076 (ft/nm) and dividing by 60 (hr/min), so with GS in knots, we get VS=tan(GPA)*101*GS. Taking our example at Renton and assuming a GS of 90 kts, this gives us VS=tan(4.2)*101.3*90=670 FPM.
Ultimately we need a rule that uses GS and GPA as inputs, and spits out a decent approximation of VS. Dividing a set of common values yields VS=k*GPA*GS, where k reliably ends up around 1.76 or 1.77. That's a bit hard to remember, so let's genericize it a bit:
Rule: VS=1.8*GPA*GS
For common examples, that gets us within 10-20 FPM, which is less error than our instruments show anyway. Any time I see a plate with non-standard glide paths, I annotate the target VS (rounded to the nearest 10 FPM) based on some ground speed inputs (to account for headwinds) and call attention to the non-standard glide path:
One approach that catches some people off guard on the descent is the RNAV 16Z into Renton. It's got a 4.2 degree glide path, so any time someone's coming in and pulls power to where they'd want it for a 3-degree path, they're going to end up high, and then sometimes chase the diamond to get back on glide. With that steep approach, that can mean exceeding 1000 FPM in the descent. If we use the equation VS=tan(GPA)*GS, we can figure out what our VS should be, and then make a rule. We need to convert from knots to FPM, which means multiplying by 6076 (ft/nm) and dividing by 60 (hr/min), so with GS in knots, we get VS=tan(GPA)*101*GS. Taking our example at Renton and assuming a GS of 90 kts, this gives us VS=tan(4.2)*101.3*90=670 FPM.
Ultimately we need a rule that uses GS and GPA as inputs, and spits out a decent approximation of VS. Dividing a set of common values yields VS=k*GPA*GS, where k reliably ends up around 1.76 or 1.77. That's a bit hard to remember, so let's genericize it a bit:
Rule: VS=1.8*GPA*GS
For common examples, that gets us within 10-20 FPM, which is less error than our instruments show anyway. Any time I see a plate with non-standard glide paths, I annotate the target VS (rounded to the nearest 10 FPM) based on some ground speed inputs (to account for headwinds) and call attention to the non-standard glide path:
Knowing what the target descent rate should be also helps smooth out corrections better than chasing the glide path diamond. If you're a bit high and you know you should be at 600 FPM, maybe try 650-700 until you start to bring it back in, then re-intercept the target value. This practice is also good for flying CDFA approaches more precisely if there's no vertical guidance.